Draw a Circle and Label All the Parts

What are the Parts of a Circle

So far, nosotros have discussed about the triangle and quadrilateral that accept linear boundaries. Circle is a closed figure that has a curvilinear boundary.
When we remember of circles, the very first affair that comes to our mind is its circular shape, for instance, bangles, coins, rings, plates, chapattis, pizzas, CDs etc. Wheels of a car, bus, cycle, truck, train, and aeroplane are likewise round in shape. If we accept a stone, necktie information technology to 1 terminate of a string and swing it in the air by holding the other end of the cord, the path traced past the stone volition be a circular path and it will make a circle.

Read More:

• Perimeter of A Circumvolve
• Common Chord of Two Intersecting Circles
• Construction of a Circle
• The Surface area of A Circle
• Properties of Circles
• Sector of A Circle
• The Area of A Segment of A Circle
• The Area of A Sector of A Circumvolve
• Classification of triangles

1. Circle: A circle is a collection of all those points in a plane that are at a given constant altitude from a given stock-still point in the airplane.
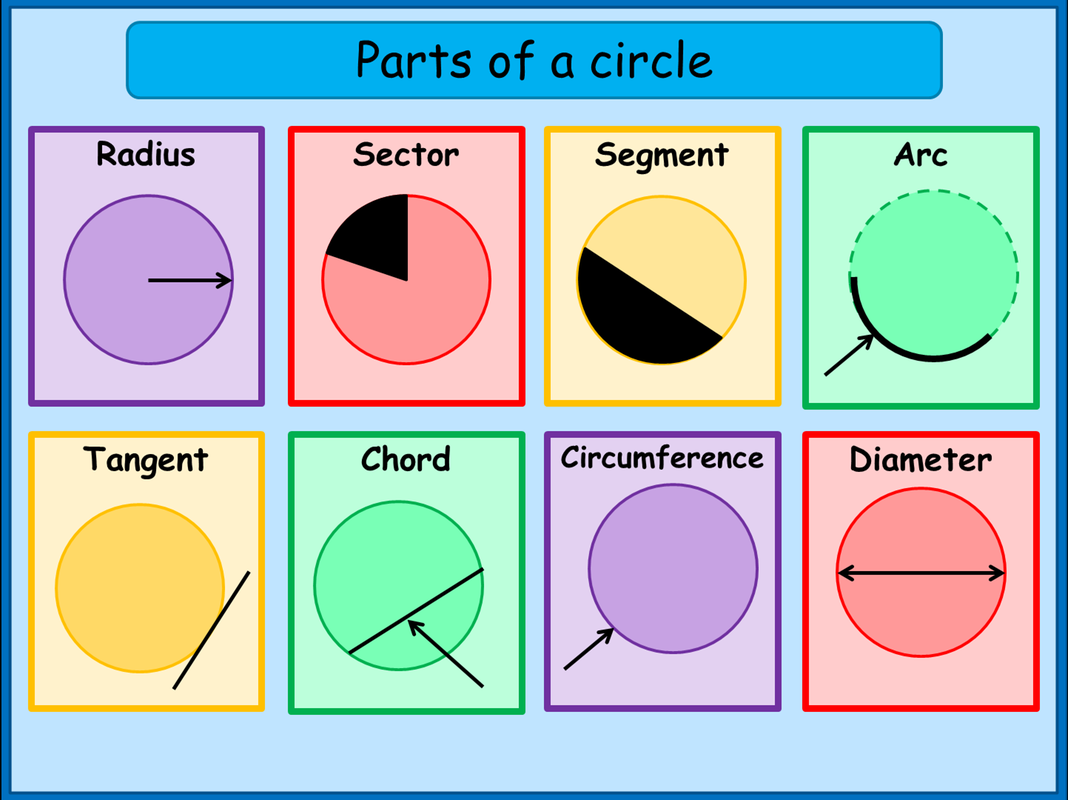
2. Eye: Circumvolve is a closed figure fabricated upward of points in a aeroplane that are at the aforementioned distance from a fixed betoken, called the center of the circle. In the figure O is the middle.
   

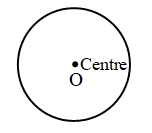
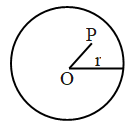
1. Radius: The abiding altitude from its eye is called the radius of the circle. In the figure, OA is radius
   
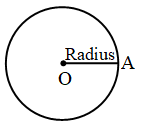
2. Chord:A line segment joining 2 points on a circle is called a chord of the circumvolve. In the figure, AB is a chord of the circle. If a chord passes through centre so it is longest chord. PQ, PR, and ST are chords of the circle. Chord ST passes through the centre, hence it is a diameter.
   
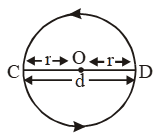
3. Diameter: A chord passing through the eye of a circle is chosen the bore of the circle. A circumvolve has an infinite number of diameters. CD is the diameter of the circle as shown in the figure. If d is the diameter of the circle then d = 2r. where r is the radius. or the longest chord is called diameter.
   In the figure, AB is the diameter and the arcs CD and DC are semicircles.
   
4. Arc: A continuous piece of a circle is called an arc. Let A,B,C,D,East,F be the points on the circle. The circle is divided into different pieces. Then, the pieces AB, BC, CD, DE, EF etc. are all arcs of the circle.
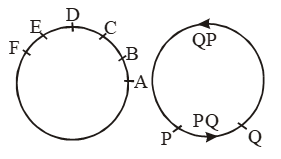   Let P,Q be two points on the circle. These P, Q divide the circle into two parts. Each part is an arc. These arcs are denoted in anti-clockwise direction
   
5. Circumference of a circumvolve:The perimeter of a circumvolve is chosen its circumference. The circumference of a circle of radius r is 2πr.
6. Semicircle: The diameter of a circle divides the circle into two equal parts. Each part is called a semi-circumvolve. We tin also say that half of a circle is chosen a semi¬circle. In the figure,  AXB and AYB represents two semi-circles.
7. Segment: Let AB be a chord of the circle. So, AB divides the region enclosed by the circle (i.eastward., the circular disc) into two parts. Each of the parts is called a segment of the circle. The segment, containing the modest arc is called minor segment and the segment, containing the major arc, is chosen the major segment and segment of a circle is the region between an arc and chord of the circle.
   
8. Central Angles: Consider a circle. The angle subtended by an arc at the heart O is called the central angle. The vertex of the central angle is e'er at the middle O.
   
9. Degree measure of an arc: Degree measure of a minor arc is the measure of the primal angle subtended by the arc.
   
   The degree mensurate of the circumference of the circle is ever 360°.
10. Interior and Exterior of Circumvolve
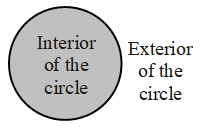
    A circumvolve divides the aeroplane on which lies into three parts.
    (i) Inside the circle. which is called the interior of the circle
    (two) Circle
    (iii) Outside the circle, which is called the exterior of the circumvolve.
    The circumvolve and its interior make up the circular region.
    
11. Sector:
    A sector is that region of a circular disc which lies between an arc and the 2 radii joining the extremities of the arc and the eye. OAB is a sector as shown in the figure.
    Quadrant: One fourth of a round disc is called a quadrant.
    
12. Position of a bespeak:
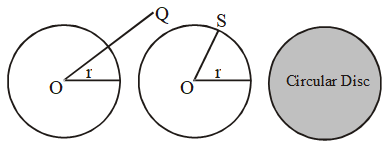
    Point Inside the circle: A bespeak P, such that OP < r, is said to lie inside the circumvolve.
    The point inside the circumvolve is also called interior point. (Example : Centre of cirle)
    Point outside the circle: A bespeak Q, such that OQ > r, is said to lie outside the circle C (O, r) = {X, OX = r}
    The signal outside the circumvolve is besides chosen exterior indicate.
    Point on the circle: A bespeak S, such that OS = r is said to prevarication on the circle C(O, r) = {X ,OX = r}.
    Circular Disc: It is defined as a set of interior points and points on the circle. In fix notation, it is written equally : C(O, r) = {X : P OX ≤ r}
    
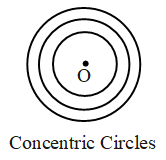
13. Concentric Circles:
    Circles having the same centre and unlike radius are said to be concentric circles.
    Remark. The word 'radius' is used for a line segment joining the center to any point on the circle and also for its length.
    
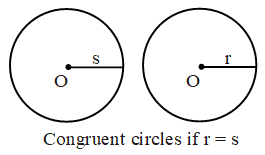
14. Congruence of Circles & Arcs
    Congruent circles: Two circles are said to be coinciding if and only if, one of them can be superposed on the other, and then as the cover it exactly. Information technology means ii circles are congruent if and simply if, their radii are equal. i.due east., C (O, r) and C (O' , r) are congruent if only if r = s.
    Congruent arcs: Two arcs of a circumvolve are congruent, if either of them can exist superposed on the other, so as to cover it exactly. Information technology is but possible, if caste measure of two arcs are the same.

Case 1: Accept ii points A and B on a aeroplane sheet. Describe a circle with A equally a middle, Air conditioning equally radius and B in its exterior.
Solution: Marker two points A and B on a newspaper.
A •            • B
Equally the indicate B should be in the outside of the circumvolve, take A as the centre and radius (r) less than AB to draw a circle.

Example 2 :Discover the diameter of the circle of radius half dozen cm.
Solution: Nosotros know,
Bore = two × radius
∴ Diameter =2 × six cm =12 cm

franklinthemblent.blogspot.com

Source: https://www.aplustopper.com/parts-of-the-circle/

Share this post